Another thing I rather fell behind on with my move to Korea is my academic reading. So I only recently got around to a short and interesting paper from August on interacting dark matter (DM).1 This is one of those papers that takes a shockingly simple idea, the kind that you look at afterwards and wonder why you didn't think about it. But it should make it easier to address a long-standing and fairly perplexing puzzle in dark matter physics.
First, let me explain the problem. DM is believed by most physicists to be one or more new, so far undiscovered particles.2 The simplest assumption to make is that DM is collisionless: the non-gravitational interactions between particles are so weak and so short-ranged that we can ignore them. This idea is also supported by the most popular top-down theoretical ideas, WIMPs and axions. This is to be contrasted with interacting DM, which refers to models where the non-gravitational couplings can not be neglected.
The collisionless assumption is very important: it makes it feasible to perform numerical studies of the galaxy formation. These start with DM being almost uniformly spread out through space, and track how tiny initial random fluctuations grow under gravity to produce galaxies, clusters of galaxies and other impressively huge things. You get beautiful pictures like this:
First, let me explain the problem. DM is believed by most physicists to be one or more new, so far undiscovered particles.2 The simplest assumption to make is that DM is collisionless: the non-gravitational interactions between particles are so weak and so short-ranged that we can ignore them. This idea is also supported by the most popular top-down theoretical ideas, WIMPs and axions. This is to be contrasted with interacting DM, which refers to models where the non-gravitational couplings can not be neglected.
The collisionless assumption is very important: it makes it feasible to perform numerical studies of the galaxy formation. These start with DM being almost uniformly spread out through space, and track how tiny initial random fluctuations grow under gravity to produce galaxies, clusters of galaxies and other impressively huge things. You get beautiful pictures like this:
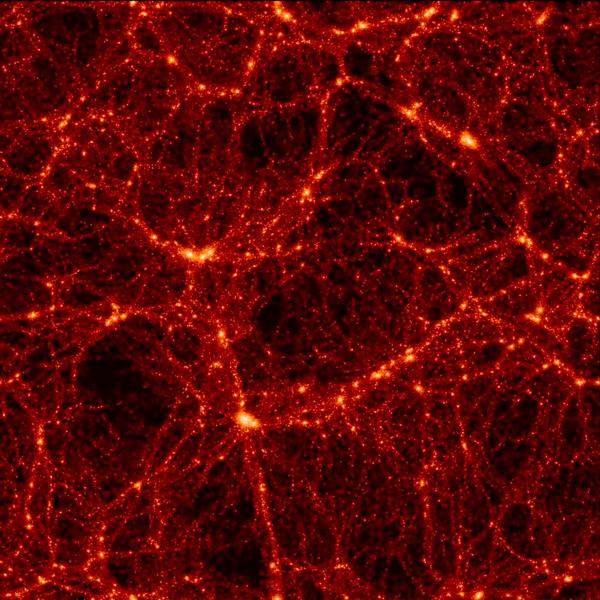 |
| Numerical simulation of DM density distributions, from the Max Planck Institute. Bright regions have high DM densities. |
Why would we want to consider interacting DM? Well, the answer is that there are hints of disagreement between the simulation results and observations. The simulations have very high DM density at the centres of galaxies, but when we look for the gravitational influence of that density spike we don't see it. For a long time it was thought that including the effects of ordinary matter might be the answer. However, this tension between theory and observation persists even for "dwarf galaxies", which have very little non-dark matter. While the case is still not completely settled, it is looking harder and harder to explain this by conventional DM physics.
If numerical simulations are problematic for interacting DM, how can we estimate its affect on structure? That's where this paper comes in. The had two simple ideas. The first was to treat interacting DM in two regimes. When the dark matter density is small, out on the edges of galaxy, the particles will interact only rarely. In this case, the usual non-interacting distributions should be a good approximation. In contrast, near the centre of the galaxy the density is high and the DM will be in approximate thermal equilibrium.
 |
| Sketch of the two regions of dark matter in a galaxy. |
The second insight in this paper is how they find the matching radius. You see, all previous studies of interacting DM used the ratio of the cross section σ (interaction strength) to the DM mass M to quantify the interactions and estimate constraints. Kaplinghat, Tulin & Yu point out that this is a poor estimate if the interaction strength varies with DM speed, which it nearly always does. Instead, they use the cross section times velocity, averaged over the galaxy, <σv>. This will be mainly determined by the cross section at the typical speed of DM in the galaxy.
This lets us determine the boundary because <σv> corresponds to the rate of interactions. Multiply this by the density of DM particles and the age of the galaxy, and you get the average number of interactions of a DM particle. Kaplinghat, Tulin & Yu chose to make 1 interaction the dividing point: inside (outside) the boundary, DM particles have scattered on average at least (no more than) once. While you could argue for a different number, this is a pretty sensible quality to use as the dividing line.
The other advantage of using <σv> is that it makes it easier to compare different objects in the Universe. For example bigger objects like clusters of galaxies tend to correspond to faster-moving DM. It is difficult to compare the results from dwarf galaxies, galaxies and clusters when working in terms of σ/m, especially when they disagree. In contrast, objects on different scales map out points in the typical velocity vs <σv>/m plane, which can then easily be compared to a candidate model. That is what is done in the following figure from this paper:
Here, the coloured points are data from different types of objects; dwarf galaxies in red, galaxies in blue and clusters in green. The preferred interaction strengths were computed using the split into non-interacting and thermal regions described above. The grey points are from calculations for velocity-independent interactions, which generally follow the straight lines. We can see that they agree well with the slower-moving objects, but quite poorly with the cluster data. The orange line is the result for a simple theory of interacting DM, with a force mediated by a light but massive state. As we can see, at high energies the interaction strength weakens, giving a better fit to the data.
The main take-away point is that by combining a simple way of modelling interacting DM with a better expression of the interaction strength, you can estimate what interaction strengths are allowed/preferred in a computationally more efficient way. And, by plotting interaction strengths as a function of mean velocity you separate out the information provided from different scales in a more useful way.
1 Well, I say recently, I've been working on this draft for a month.↩


No comments:
Post a Comment