Just because something is infinite does not mean it is zero!The infinities here show up in quantum field theory (QFT) when we look at the perturbation series beyond leading order. They confused people a lot during the development of QFT, but their resolution is physically interesting, and something I've been meaning to talk about for a while. Today, I'm only going to be able to set up the problem; I hope to get to the solution by the end of the week.
Let's start by remembering that Feynman diagrams are an easy way to represent a perturbation series. All the Feynman diagrams I've shown so far have been tree-level: they have been without loops.
 |
| Some example tree-level Feynman diagrams; from 1211.0961 |
For example, assume that all vertices in our theory involve three lines, as in the diagrams above. The simplest possible diagram contains three external lines, one vertex and no internal lines:
We know all external momenta, and overall conservation momentum is enforced by its conservation at the vertex. Now add a vertex to this diagram. The only way to do this without making a loop is to add it to the end of one of the external lines, making it an internal line and adding two new external ones.
We already knew the momenta on line 2, and the overall conservation momentum follows from it at the new vertex. By continually adding to diagrams like this, we can construct all possible tree diagrams, and see that if there are no loops then there are no unknown momenta.
This changes once we consider diagrams with loops. As the simplest example, consider the following:
Overall conservation of momentum requires that the momenta on the two external lines be the same, labelled here by P. It then follows that for any choice for the loop momentum L, we can satisfy conservation of momentum at both vertices.
We can think of each different value of the loop momentum as being a slightly different process. Since QFTs are quantum theories, we add all the different processes together to get the overall theoretical result. Slightly more formally, we integrate over all possible values of the loop momentum. And it's here that we start to get problems.
To focus our discussion, let's consider a specific process: electron-positron annihilation to a pair of muons. This is a standard introductory example for QFTs, but also has real applicability to calibrating collider experiments including the LHC. At low energies, there is one tree diagram:
Using this diagram to predict the rate for this process agrees with experiment to the percent level or better, including not only the overall rate but as a function of energy, or the muon angle. We would estimate the theoretical error as the size of the fine structure constant, 1/137. The agreement between theory and experiment tells us we must have something fundamentally right.
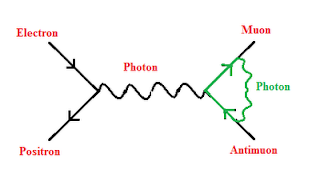
What are the theoretical corrections to this? There are eight diagrams at the next order in perturbation theory with the same initial and final states. We don't need to go into all of them, however; just one will suffice:
We have added a photon connecting the two final state muons. We will have to integrate over the energy and momentum of this photon.
The first problem we find comes when the new photon has equal energy and momentum (in natural units). The amplitude for the loop diagram includes a piece
$M \sim \frac{1}{E_\gamma^2 - p_\gamma^2 c^2}$
So when the photon energy E equals p c (with c the speed of light) we get one over zero. This is undefined, or colloquially, infinity. Infinities of this kind are known as infrared divergences.The other problem arises when the photon has very large energy and/or momentum. Let's assume the energy is large. The photon energy also determines the energy of the two internal muons shaded green in the figure above; if the photon energy is large, they also have large energy. In this limit, the amplitude looks like
$M \sim \frac{1}{E_\gamma^4}$
We get two factors of energy in the denominator from the photon line, and one each for the two muons. At first sight, this doesn't look problematic; after all, it goes to zero as the energy becomes large. The problem is that we have to sum over an infinite number of possibilities. We have four possible numbers that can get large -- the energy and the three components of the momentum -- so the number of states with at least one of those numbers large goes like the cube of the energy. (Consider the surface of a sphere; this is proportional to the square of its radius. With four-vectors, we are interested in hyper-spheres in four dimensions; their surface varies as the radius cubed.) This leaves us with the rough estimate
$M \sim \int E^3 dE \frac{1}{E^4},$
which is infinite. Problems of this type are called ultraviolet divergences, as they arise from high energies in the loop.Let's review. The first term in our perturbation series makes sense, agreeing with experiment to the expected level. This tells us something is right. But our second term in the perturbation series is nonsense; we get infinities as predictions for finite quantities, telling us that something is badly wrong. The original approach was to throw the infinities away. Here we see the difference between a theoretical physicist and a mathematician; the latter would be worried about whether our calculation means anything, while the physicist takes the pragmatic approach that if we can get accurate predictions don't worry about the nonsensical part.
However, the need for a deeper understanding became clear when Hans Bethe (inspired by Schwinger and Weisskopf) "subtracted" two infinities to get a finite result, a calculation of the Lamb shift. It is in this background that Weinberg's quote originated; the infinities are not just garbage, but contain actual physics. What that physics is will have to wait for a later post.





No comments:
Post a Comment